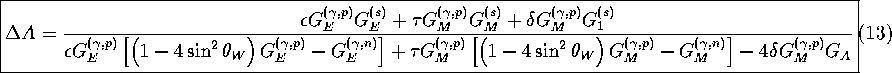Département de Physique Nucléaire

Institut de recherche sur les lois fondamentales de l'Univers

Introduction

Due to the high mass of the , elastic eP scattering is dominated at low energy by photon exchange. In order to measure the neutral weak form factors in elastic electron proton scattering, one needs to be able to separate the electromagnetic contribution from the weak one . One way would be to run at higher energy, but then the elastic eP cross section falls off to rapidly. Using Polarized electron enables to take benefit of the fact that the weak interaction does not conserves parity, thus a Left-Right asymmetry will gives access to

Why Parity Violation with Polarized electron

 
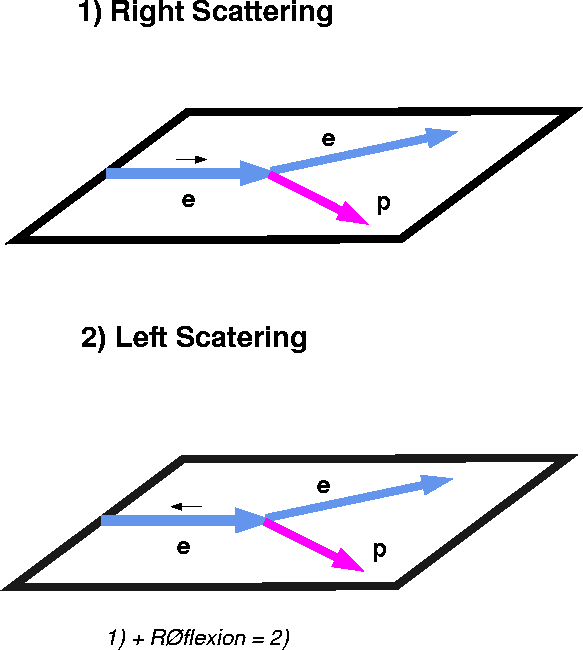
Figure 1: A reflexion wrt to the scattering plane (x,y) ., transform elastic right electron-proton scattering to left electron-proton scattering.

Using longitudinally polarized electrons, an asymmetry between left and right (longitudinal polarization) electron for elastic scattering on unpolarized proton is the manifestation of an interaction which is not invariant by reflexion wrt the scattering plane (x,y) . The parity is a product of this reflexion with a rotation of along the axis perpendicular to the mirror plane

Since Physics is invariant under rotation, this is equivalent to an interaction not invariant under parity.

Asymmetry versus

The asymmetry between scattering with Right and Left electron is

This asymmetry is plotted on figure (2), assuming no strange quarks, no evolution for and using the Simon's and Beise's fit for the nucleon EM form factors. Also given the relative extra asymetry with stange quark as predicted by jaffe ,Weigel and souder (figure 3). The asymmetry can also be written using Sachs Form factors :

with

The asymmetry is very little sensitive to the axial form factor , because of the axial coupling of the electron with quark is killed by the term. A better way to measure is via neutrino proton scattering. In our case, the asymmetry is thus sensitive to and .

 
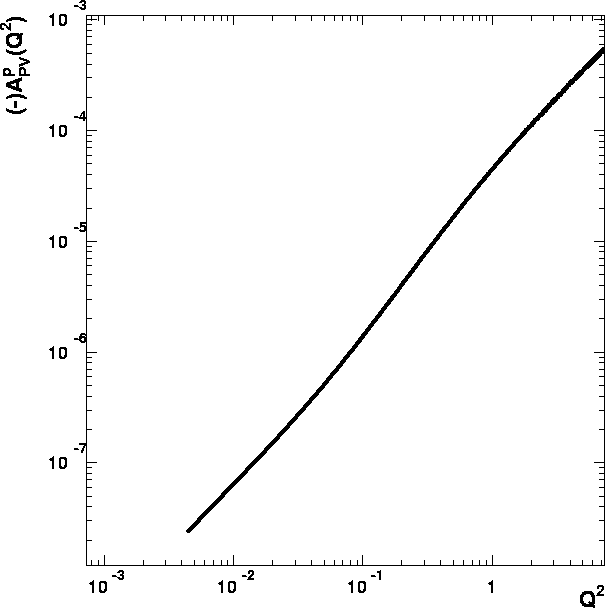
Figure 2: The asymmetry for proton elastic scattering with Right and left electrons, assuming no strange quarks, for E=4.35 GeV, with , using Beise parametrization. 

 
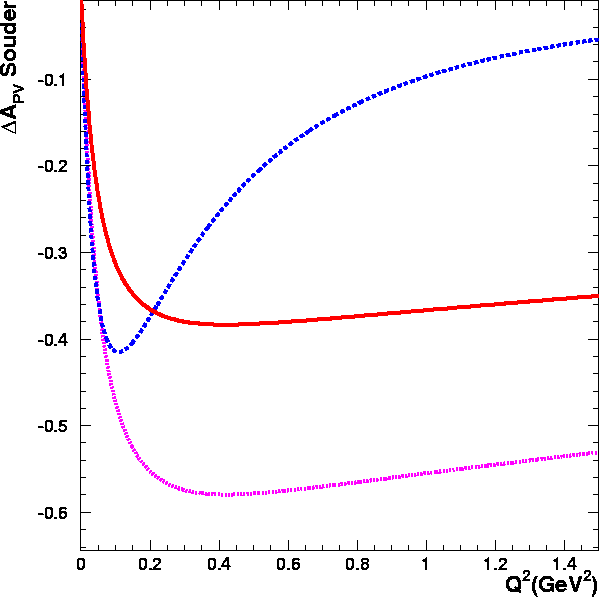
Figure 3: The relative extra asymmetry for proton elastic scattering with Right and left electrons, with strange quarks using Jaffe/Souder predictions 

To see which combination of the form factors will be measured, let be the asymmetry expected for no strange quark in the proton, using (see Eq. )

(see Eq. )

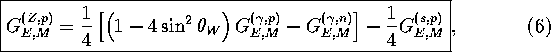
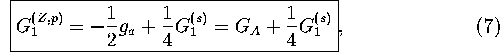
one gets

with

and

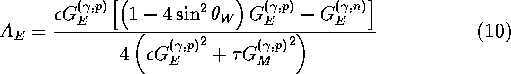
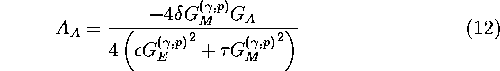
The additional asymmetry due to strange quark is