Chapter 4
Modeling Cosmic Dust Evolution

This chapter focusses on the study of dust evolution in all interstellar environments, at all spatial scales. Dust evolution is the variation of the constitution of a grain mixture with time, under the effects of its environment. The timescales of evolution being significantly longer than the career of a scientist, we usually study spatial variations of the dust content in a region, or the variations among a sample of galaxies. These different observations are then compared, being considered as snapshots at different evolutionary stages. The environmental parameters that are commonly used to quantify dust evolution are: (i) the ISM density and the ISRF intensity and hardness, for spatially-resolved studies; (ii) the metallicity and star formation rate, for global galactic studies. The main processes responsible for dust evolution are represented on Fig. 4.1.

Grain Formation

is the dust mass build-up by:

• grain condensation in the ejecta of core-collapse SNe and AGB stars (i.e. making grains from scratch by condensing atoms);
• grain (re-)formation in the CNM and molecular clouds, by accretion of atoms and molecules onto grains: (i) grain growth; (ii) mantle accretion; and (iii) ice formation.

Grain Processing

is the alteration of the grain constitution in the ISM by:

• shattering and fragmentation by grain-grain collisions in low-velocity shocks (modification of the size distribution);
• structural modifications by high energy photons or cosmic ray impacts;
• grain-grain coagulation in cold regions.

Grain Destruction

is the full or partial removal of the elements constituting the grains by:

• erosion and evaporation by thermal or kinetic sputtering (gas-grain collision in a hot gas or a shock);
• photodesorption of atoms and molecules;
• thermal evaporation;
• astration (incorporation into stars).

4.1 Stellar Evolution

Stars have a crucial impact on ISD: (i) they synthesize the heavy elements that constitute dust grains (Fig. 2.16); (ii) they also directly produce dust seeds in their ejecta; (iii) the shock waves of SNe erode and vaporize the grains; (iv) the radiative and mechanical feedback of massive stars carve the ISM and process the grains.

4.1.1 The Fate of Stars of Different Masses

A star can be conceptualized as a sphere of gas in hydrostatic equilibrium, where the gravity is counterbalanced by the thermal pressure sustained by nuclear reactions in its core (e.g. Degl’Innocenti, 2016, for an introduction). The energy produced in the core is carried out through radiation, convection or conduction. The initial mass of a star, and in a lesser extent its initial metallicity, determine its future evolution.

4.1.1.1 Nucleosynthesis

The nuclear reactions in stellar interiors, on top of being the fuel of stars, lead to the production of heavy elements. A fraction of these freshly synthesized elements are injected back into the ISM, during the final stages of stellar evolution.

Nuclear binding energies. A fundamental quantity to determine the efficiency of nuclear reactions to sustain the thermal pressure within a star is the nuclear binding energy of an element of mass A (number of nucleons; cf. e.g. Chaps. 1-2 of  Pagel, 1997, for a review). This quantity is represented in Fig. 4.2 for the most relevant nuclei. To have an exothermic reaction, that will be able to counterbalance gravity, one needs to synthesize elements of higher binding energies. The curve of Fig. 4.2 reaches a maximum around ${}^{56}$Fe.

Fusion

of elements lighter than ${}^{56}$Fe is exothermic. Since the initial composition of a star is $\simeq 3∕4$ H and $\simeq 1∕4$ He, stellar nucleosynthesis takes this way.

Fission

of elements heavier than ${}^{56}$Fe is exothermic. This is the process implemented in nuclear reactors to generate electricity (through heat).

Primordial nucleosynthesis. Before the first stars appeared, ${}^2$H and ${}^4$He, as well as elements up to ${}^7$Li, were synthesized during the first 15 minutes after the big bang (e.g. Pagel, 1997; Calura & Matteucci, 2004; Johnson, 2019). The temperature was at this time around $T\simeq 10^9$ K. This primordial nucleosynthesis was brief, as the Universe was expanding and cooling. It is estimated that after 20 minutes, the temperature was too low to synthesize new elements. The primordial abundances refer to the elements produced during these first minutes (cf. Eq. $2.11$):

Stellar nucleosynthesis. Once the temperature at the center of a collapsing protostar becomes high enough ($T\simeq 10^7$ K), thermonuclear reactions ¹ are initiated. Several chains and cycles of reactions occur in stars, at different stages. The most important ones are the following (e.g. Filippone, 1986; Pagel, 1997; Silva Aguirre, 2018).

Proton fusion,

also called p-p chain, is a series of nuclear reactions converting 4${}^1$H into ${}^4$He. This reaction chain has three branches cycling through various light elements (D, Li, B, Be). It is the dominant process in stellar interiors with $T\lesssim 2\times 10^7$ K, that is for stars with mass $m_{\star }\lesssim 1M_{\odot }$. ²

CNO cycle

is another series of nuclear reactions converting 4${}^1$H into ${}^4$He. Contrary to the p-p chain, this cycle requires pre-existing C, N or O (i.e. it requires a non-zero-metallicity star). This cycle can be broken into: (i) a CN cycle, starting with ${}^{12}$C and 4${}^1$H, ending with ${}^{12}$C and ${}^4$He; and (ii) a NO cycle, starting with ${}^{15}$N and 3${}^1$H, ending with ${}^{14}$N and ${}^4$He. This cycle is more efficient than the p-p chain for $T\gtrsim 2\times 10^7$ K, that is for stars with $m_{\star }\gtrsim 1M_{\odot }$. In practice, both happen simultaneously, but with different efficiencies.

The triple $\alpha$ process

is a series of nuclear reactions converting 3${}^4$He into ${}^{12}$C (the ${}^4$He nucleus is indeed called the $\alpha$ particle). It also produces ${}^{16}$O and ${}^{20}$Ne as byproducts. This process starts when the star has converted $\simeq 10\%$ of its H into He. It requires temperatures of $T\simeq 10^8$ K.

Heavier element fusion

happens essentially in massive stars ($m_{\star }\gtrsim 8M_{\odot }$), when the temperature of the core reaches $T\simeq 10^9$ K. Several successive phases are then possible: C burning, Ne burning and O burning, producing up to ${}^{28}$Si. The last series of reactions are the $\alpha$ ladder, which produce elements up to Fe and Ni.

4.1.1.2 Brief Outline of Stellar Evolution

Stars are born from the collapse of molecular clouds into protostars (e.g. Motte et al., 2018, for a review). Protostars accrete matter until their winds and radiation pressure stops this process, leading to a pre-main sequence star (pre-MS). Pre-MS stars exhibit violent winds and bipolar jets, clearing away the remaining molecular cocoon they were born in. They contract until the temperature in their core is high enough to initiate H fusion ($T\simeq 10^7$ K). Below $m_{\star }\le 0.08M_{\odot }$, we get a brown dwarf , which is a compact object not massive enough to sustain nuclear reactions. Fig. 4.3 schematically represents the different stages of evolution of low- and high-mass stars.

The Main Sequence. Once nuclear reactions ignite, stars are on the Zero-Age Main Sequence (ZAMS; grey line in Fig. 3.18). We have already briefly discussed stellar evolution in Sect. 3.1.2.3. The different types of stars, their mass, luminosities and lifetimes are given in Fig. 3.18 and in Table 3.2.

1.

As long as stars are in their H burning phase (cf. Sect. 4.1.1.1) they are Main Sequence (MS) stars. They move slowly along their tracks in Fig. 3.18.

2.

Once the core has exhausted its H, it contracts by lack of fuel. This contraction increases the temperature, allowing the He burning to start (cf. Sect. 4.1.1.1). Due to the increase of central temperature, the outer layers expand. The star is now a red giant.

3.

This process repeats, with He burning (triple $\alpha$ process; Sect. 4.1.1.1).

The late stages of massive stars. Massive stars ($8M_{\odot }\le M_{\star }<120M_{\odot }$) are the hottest and most luminous ones (cf. Table 3.2). They are short-lived ($\tau \left(m_{\star }\right)\lesssim 30$ Myr; Fig. 3.18).

1.

The process of burning heavier elements is repeated beyond C, resulting in an “onion” structure (cf. Fig. 4.3.a). The combustion of each element is exponentially faster. These stars are, at this point, red supergiants (cf. Fig. 3.18).

2.

Once the core is made of Fe, the star can not anymore produce energy by nuclear fusion. It therefore collapses (e.g. Heger et al., 2003).

If $m_{\star }\lesssim 40M_{\odot }$,

the collapse is halted by the degeneracy pressure ³ of neutrons. The outer layers of the stars then explode as a type II supernova (SN II) or core-collapse supernova, leaving a Neutron Star (NS) in the center.

If $m_{\star }\gtrsim 40M_{\odot }$,

the degeneracy pressure of the neutron core is not sufficient to sustain the collapse. The remnant is not anymore a NS, but a Black Hole (BH). This is also the approximate mass range where the star leaves a remnant without exploding as a SN, ending as a collapsar (Heger et al., 2003). These two phenomena (ending as a collapsar and leaving a BH) are not necessarily concomitant. The exact masses above which these two phenomema occur are not accurately known and depend on other parameters, such as stellar rotation. For simplicity, we have represented both phenomena on the same branch in Fig. 4.3.

The late stages of LIMS. Low- and Intermediate-Mass Stars (LIMS; $0.08M_{\odot }\le M_{\star }<8M_{\odot }$) are less luminous than massive stars, but they are the most numerous. Their lower gravity allow them to burn their elements slower than massive stars, and therefore to live longer (several Gyrs, on average; Fig. 3.18).

1.

Their mass does not allow them to start C burning. LIMS enter the Asymptotic Giant Branch (AGB; cf. Fig. 3.18; e.g. van Winckel, 2003; Herwig, 2003, for reviews). They are larger and more luminous than red giants and are thermally pulsing.

2.

The contraction of the core is halted by electron degeneracy pressure. The thermal pulses lead the outer shell to expand progressively, creating a Planetary Nebula (PN), leaving a White Dwarf (WD) in the center.

3.

The maximum mass a white dwarf can reach is the Chandrasekar mass, $m_{\mathrm{Chandra}}\simeq 1.4M_{\odot }$. If a white dwarf of mass $m_{\mathrm{WD}}$ happens to be in a binary system with another red giant, it will accrete some of its mass.

If $m_{\mathrm{WD}}\gtrsim m_{\mathrm{Chandra}}$,

the excess of mass above the Chandrasekar limit re-ignites the thermonuclear reactions. It follows a type Ia SN (SN Ia), which disrupts the binary system.

If $m_{\mathrm{WD}}\lesssim m_{\mathrm{Chandra}}$,

thermonuclear reactions are ignited at the surface of the white dwarf. It ensues a nova, that does not disrupt the binary system.

4.1.1.3 Parametrizing Star Formation

Star formation is a complex process involving stars of different masses being formed at different times. At the scale of a star-forming region or an entire galaxy, SF can be described statistically.

Initial mass functions. Initial Mass Functions (IMF) express the number distribution of stars of mass $m_{\star }$ born at a given time: $\varphi \left(m_{\star }\right)\equiv d{N}_{\star }∕d{m}_{\star }$. IMFs are usually expressed in $M_{\odot }^{-1}$, and are normalized as ⁴:

where $m_{-}=0.1M_{\odot }$ and $m_{+}=100M_{\odot }$ are the lower and upper masses. The average stellar mass is defined as:

The fraction of stars ending their life as a core-collapse SN is:

with $m_{-}^{\mathrm{SN}}=8M_{\odot }$ and $m_{+}^{\mathrm{SN}}=40M_{\odot }$ (e.g. Heger et al., 2003). Several IMFs have been proposed in the literature (see also  Kroupa, 2001).

The Salpeter IMF

(Salpeter, 1955) was the first one proposed. It is defined as:

$${\varphi }_{\mathrm{Salp}}\left(m_{\star }\right)\equiv \frac{\left(1-\alpha \right)\times m_{\star }^{-\alpha }}{m_{+}^{1-\alpha }-m_{-}^{1-\alpha }},\text{ with }\alpha =2.35$$

(4.5)

where the lower and upper masses, $m_{-}$ and $m_{+}$, are usually taken as $m_{-}=0.1M_{\odot }$ and $m_{+}=100M_{\odot }$, although the original Salpeter (1955) study constrained the index of the power-law only up to $m_{+}=10M_{\odot }$.

The Chabrier IMF

(Chabrier, 2003) for individual stars is defined, within the same mass range, as:

$${\varphi }_{\mathrm{Chab}}\left(m_{\star }\right)\equiv \left\{\begin{array}{cc}0.16033\times m_{\star }^{-2.3}\hfill & \text{ for }{m}_{\star }>1M_{\odot }\hfill \\ \frac{0.5718}{m_{\star }}\exp <!--\; FUNCTION\; APPLICATION\; -->\left(-\frac{{\left({\log <!--\; FUNCTION\; APPLICATION\; -->}_{10}\left(m_{\star }\right)+1.1023729\right)}^2}{0.9522}\right)\hfill & \text{ for }{m}_{\star }\le 1M_{\odot }.\hfill \end{array}\right.$$

(4.6)

The top-heavy IMF

(e.g. Dwek et al., 2007) is often invoked at high-redshift. It is defined as:

$${\varphi }_{\mathrm{Top}}\left(m_{\star }\right)\equiv \frac{\left(1-\alpha \right)\times m_{\star }^{-\alpha }}{m_{+}^{1-\alpha }-m_{-}^{1-\alpha }},\text{ with }\alpha =1.50$$

(4.7)

These IMFs are compared in Fig. 4.4. Some of their properties are listed in Table 4.1. The IMF is thought to be a universal property of interstellar media. The different IMFs of Table 4.1 have consequences on the stellar properties. The current consensus is that the Chabrier IMF might be more appropriate than Salpeter’s, at least at low redshift.

Parametric star formation histories. We have already briefly discussed Star Formation Histories (SFH) in Sect. 3.1.2.3. The SFH quantifies the SFR, $\psi \left(t\right)$, as a function of time, $t$, of a star-forming region or galaxy. Several parametric forms are commonly used in the literature. As we will see in Sect. 4.3, their parameters can be inferred by fitting a set of observations.

The exponential SFH

(cf. Fig. 4.5.a) is parametrized by a timescale, ${\tau }_{\mathrm{SF}}$, and a SFR at $t=0$, ${\psi }_0$:

$${\psi }_{\exp }\left(t\right)\equiv {\psi }_0\exp <!--\; FUNCTION\; APPLICATION\; -->\left(-\frac{t}{{\tau }_{\mathrm{SF}}}\right).$$

(4.8)

The delayed SFH

(cf. Fig. 4.5.b; Lee et al., 2010) has the same number of parameters as the exponential SFH, but its SFR peak is delayed at $t={\tau }_{\mathrm{SF}}$:

$${\psi }_{\mathrm{del}}\left(t\right)\equiv {\psi }_0\frac{t}{{\tau }_{\mathrm{SF}}}\exp <!--\; FUNCTION\; APPLICATION\; -->\left(-\frac{t}{{\tau }_{\mathrm{SF}}}\right).$$

(4.9)

It is possible to combine several SFHs to account for the complex history of a galaxy. A useful quantity, deriving from the SFH, is the stellar birth rate, which is the average number of stars born per unit time:

The rate of SN II, $R_{\mathrm{SN}}\left(t\right)$, can be approximated from this quantity:

where $\tau \left(m_{\star }\right)$ is the lifetime of a star of mass $m_{\star }$ (cf. Fig. 3.18). The approximation, in the second part of Eq. ($4.11$), comes from the fact that the lifetime of massive stars ($\tau \left(m_{\star }\right)\lesssim 30$ Myr) is usually much smaller than the SF timescale: $\tau \left(m_{\star }\right)«{\tau }_{\mathrm{SF}}$.

4.1.2 Elemental and Dust Yields

Stars, in their late stages, return to the ISM a fraction of the heavy elements they have synthesized. Stellar ejecta are: (i) stellar winds; (ii) planetary nebulae; (iii) novae; (iv) SN Ia; and (v)  SN II. In addition, the temperature in these ejecta can be low enough (cf. Fig. 2.17.b) to condense grains, that we refer to as stardust.

4.1.2.1 Injection of Heavy Elements in the ISM

Fig. 4.6 gives the approximate fraction of each element produced in different environments, for the Solar neighborhood (e.g. Johnson, 2019). These proportions depend on the past SFH of the system we are considering.

Primordial nucleosynthesis

(cf. Sect. 4.1.1.1) is responsible for the production of most of the light elements.

SN II

account for most of the ISD-relevant elements, except C.

AGB ejecta

(i.e. LIMS winds and PNe) account for a significant fraction of C and N, and heavier elements we have not displayed here.

SN Ia

are responsible for synthesizing a significant fraction of the metals around Fe.

Other processes,

such as cosmic ray fission and merging neutron stars, are not very relevant to ISD.

Stellar elemental yields. A stellar yield, $Y_E\left(m_{\star }\right)$, is the mass of an element E injected into the ISM by a star of mass $m_{\star }$, at the end of its lifetime. These yields can be constrained observationally, but they are essentially determined theoretically (e.g. Karakas & Lattanzio, 2014, for a review). Fig. 4.7.a shows the yields of the most important elements. An important quantity determining the type of dust grains that will form in the ejecta is the C/O ratio. Indeed, when the temperature cools down enough, C and O tend to combine to form CO molecules. The excess atom will thus be the only one left to form stardust. Therefore, stellar ejecta with $\text{C}<\text{O}$ will form primarily O-rich grains (silicates and oxides), whereas stellar ejecta with $\text{C}>\text{O}$ will form mainly carbon grains and SiC. Fig. 4.7.b compares the number abundances of C and O ejected by stars of different masses. We can see that carbon grains originate mainly in LIMS around $m_{\star }\simeq 3M_{\odot }$.

Metallicity estimates. The heavy elements injected by the successive stellar populations increase the metallicity of the ISM, Z. In that sense, the metallicity is an indicator of “the fraction of baryonic matter that has been converted into heavier elements by means of stellar nucleosynthesis” (Kunth & Östlin, 2000), such that:

The measure of metallicity is not completely straightforward and is vigorously debated (e.g. Kewley et al., 2019, for a review). In external galaxies, it is usually estimated by modeling observations of nebular optical lines, coming from H II regions. Several methods, allowing an observer to convert a few line ratios into a metallicity estimate, have been proposed. These methods have been calibrated on particular H II regions, modeling the photoionization, making unavoidable assumptions about the stellar populations and the topology of the gas. We have systematically compared several calibrations over the DustPedia sample (De Vis et al., 2019). We have favored the “S” calibration from Pilyugin & Grebel (2016), as it is the most reliable down to low metallicities.

4.1.2.2 Production of Stardust

At the scale of a galaxy, the two most important sources of stardust are: (i) AGB stars, encompassing both LIMS winds and PNe; and (ii) SN II.

AGB stars. Most of the dust production in LIMS is believed to occur during the Thermally-Pulsing Asymptotic Giant Branch (TPAGB) phase (Gail et al., 2009). In addition, LIMS with $m_{\star }\lesssim 1M_{\odot }$ do not condense grains (e.g. Ferrarotti & Gail, 2006). Theoretical models concur that only a fraction of the available heavy elements will go into stardust (Morgan & Edmunds, 2003; Ventura et al., 2012):

$m_{\mathrm{stardust}}^{\mathrm{ej}}$ and $m_Z^{\mathrm{ej}}$ being the ejected mass of stardust and heavy elements. Observations and modeling of the circumstellar envelopes of post-AGB stars are consistent with these values (e.g. Ladjal et al., 2010).

SN II. There is solid evidence that grains form in SuperNova Remnants (SNR), as the ejected gas cools down. Theoretical estimates of the net dust yield of a single SN II range in the literature from $Y_{\mathrm{SN}}\simeq 10^{-3}$ to $Y_{\mathrm{SN}}\simeq 1M_{\odot }∕\text{SN}$ (e.g. Todini & Ferrara, 2001; Ercolano et al., 2007; Bianchi & Schneider, 2007; Bocchio et al., 2016; Marassi et al., 2019). From an observational point of view, measuring the dust mass produced in situ by a single SN II is quite difficult, as it implies disentangling the freshly-formed dust from the surrounding ISM. It also carries the usual uncertainty about dust optical properties. A decade ago, the largest dust yield ever measured was $Y_{\mathrm{SN}}\simeq 0.02M_{\odot }$ (in SN$$2003gd;  Sugerman et al., 2006). The Herschel space telescope has been instrumental in estimating the cold mass of SNRs. The yields of the three most well-studied SNRs are now an order of magnitude higher:

Cassiopeia A:

$Y_{\mathrm{SN}}\simeq 0.04-1.1M_{\odot }$ (Barlow et al., 2010; Arendt et al., 2014; De Looze et al., 2017; Bevan et al., 2017; Priestley et al., 2019);

The Crab nebula:

$Y_{\mathrm{SN}}\simeq 0.03-0.23M_{\odot }$ (Gomez et al., 2012; Temim & Dwek, 2013; De Looze et al., 2019);

SN 1987A:

$Y_{\mathrm{SN}}\simeq 0.45-0.8M_{\odot }$ (Dwek & Arendt, 2015; Matsuura et al., 2015).

Most of the controversy however lies in the fact that, while large amounts could form in SN II ejecta (e.g. Matsuura et al., 2015; Temim et al., 2017), a large fraction of freshly formed grains would not survive the reverse shock ($v\simeq 1000$ km/s; e.g.  Nozawa et al., 2006; Micelotta et al., 2016; Kirchschlager et al., 2019). In all the cases we have listed above, the newly-formed grains have indeed not yet experienced the reverse shock (Bocchio et al., 2016). The net yield is thus expected to be significantly lower. Even if $\simeq 10-20\%$ of the dust condensed in an SN II ejecta survives its reverse shock (e.g. Nozawa et al., 2006; Micelotta et al., 2016; Bocchio et al., 2016), we have to also consider the fact that massive stars are born in clusters. The freshly-formed dust injected by a particular SN II, having survived the reverse shock, will thus be exposed to the forward shock waves of nearby SNe (e.g. Martínez-González et al., 2018). Overall, SN II dust yields are largely uncertain. We will extensively discuss their empirical constraint, from a statistical point of view, in Sect. 4.3. We will show that we can infer the average dust yield per SN II, $⟨Y_{\mathrm{SN}}⟩$. The corresponding timescale is then simply:

Indirect evidence. The best constraints on the fraction of ISD which is stardust might be indirect. The clear correlation between the depletion factor, $F_{\star }$, and the average density of the ISM, $⟨n_H⟩$, that we have discussed in Fig. 2.17.a, has been shown to require rapid destruction and reformation into the ISM (e.g. Draine & Salpeter, 1979; Tielens, 1998; Draine, 2009). The rest of the grains needs to form in the ISM. Draine (2009, D09) gives a series of additional arguments concluding that, in the MW, stardust has to be less than $10\%$ of ISD.

1.

A first argument given by D09 is based on the typical lifetime of ISD.

a)

From the literature, the stardust injection rate is roughly ${Ṁ}_{\mathrm{stardust}}\simeq 5\times 10^{-3}{M}_{\odot }∕\text{yr}$.

b)

Noting that the typical lifetime of a dust grain in the MW is ${\tau }_{\mathrm{ISD}}\simeq 3\times 10^8$ yr (cf. Sect. 4.2.2.3;Jones et al., 1996), the present stardust mass in the ISM should be: $M_{\mathrm{stardust}}\simeq {Ṁ}_{\mathrm{stardust}}\times {\tau }_{\mathrm{ISD}}\simeq 1.5\times 10^6{M}_{\odot }$.

c)

The ISD mass in the MW is roughly $M_{\mathrm{ISD}}\simeq M_{\mathrm{ISM}}∕183\simeq 2.7\times 10^7{M}_{\odot }$ (cf. Table 2.4).

Stardust is thus only $M_{\mathrm{stardust}}∕M_{\mathrm{ISD}}\simeq 5\%$ of the total ISD.

2.

A second argument given by D09 is based on the study of IDPs in meteorites (cf. Sect. 2.2.4).

a)

Stardust silicate grains in meteorites, identified with their isotopic anomalies (cf. Fig. 2.19), appear to be $\simeq 20\%$ crystalline.

b)

Silicates in the ISM are less than $\lesssim 2\%$ crystalline (cf. Sect. 2.2.1.2; Kemper et al., 2004).

Therefore, the fraction of stardust is only less than $\lesssim 2∕20\simeq 10\%$ of ISD. Also, the fact that ISD is mainly amorphous, whereas circumstellar grains are essentially crystalline, is another argument in favor of rapid destruction and reformation in the ISM.

4.2 Dust Evolution Processes in the ISM

Most of the important dust evolution processes occur in the ISM. These effects can be studied by looking at spatial variations of the dust properties in a region.

4.2.1 Grain Formation and Transformation

Grain formation is the transfer of elements from the gas phase to the dust phase, therefore increasing the dustiness.

4.2.1.1 Evidence of Grain Growth and Coagulation in the ISM

We have just discussed stardust (cf. Sect. 4.1.2.2) which is thought to produce grain seeds onto which mantle can grow. We now focus on the dominant process in Solar metallicity systems: the accretion of gas phase atoms and molecules. Grain-grain coagulation does not result in grain formation per se, as it does not affect the dustiness. It however follows grain growth and has similar effects on the FIR opacity.

The evidence brought by depletions. As we have discussed in Sect. 4.1.2.2, the clearest evidence of grain growth in the ISM is provided by the good correlation between the depletion factor and the average density of the ISM (cf. Fig. 2.17.a). It implies that atoms and molecules from the gas phase are progressively building up grain mantles, when going into denser regions. This observed behaviour is also consistent with the progressive de-mantling and disaggregation of cloud-formed, mantled and coagulated grains injected into the low density ISM, following cloud disruption. It is perhaps not unreasonable to hypothesise that dust growth in the ISM occurs on short timescales during cloud collapse rather than by dust growth in the quiescent diffuse ISM. In this alternative interpretation, the arrow of time is in the opposite sense and requires rapid dust growth, through accretion and coagulation, in dense molecular regions and slow de-mantling and disaggregation in the diffuse ISM (e.g. Jones, 2009). Given that astronomical observations provide only single-time snapshots, it will seemingly be difficult to determine the direction of the time-arrow of dust evolution.

FIR opacity variations. We have seen in Fig. 1.21 that the growth of mantles has an impact on the FIR opacity (cf. Köhler et al., 2014, 2015). Yet, there is clear evidence of FIR opacity variations in the MW. The main factor seems to be the density of the medium. For instance, both Stepnik et al. (2003) and Roy et al. (2013) found that the FIR dust cross-section per H atom increases by a factor of $\simeq 3$ from the diffuse ISM to the molecular cloud they targeted. Stepnik et al. (2003) noticed that this opacity variation is accompanied by the disappearance of the small grain emission. They concluded that grain coagulation could explain these variations. In the diffuse ISM, Ysard et al. (2015) showed that the variation of emissivity, including the $\beta -T$ relation (cf. Sect. 3.1.2.1), could be explained by slight variations of the mantle thickness of the THEMIS model. For that reason, the THEMIS model aims at describing the evolution of grain mantles as a function of density and ISRF, as we have seen in Fig. 1.21: (i) in the diffuse ISM, the grains are supposed to have a thin a-C mantle, largely dehydrogenated (aromatic) by UV photons; (ii) in denser regions, the mantle thickness is hypothesized to increase and to become more hydrogenated (aliphatic), because of the progressive shielding of stellar photons; (iii) in molecular clouds, grains are thought to be coagulated and iced. The THEMIS model predicts a factor of $\simeq 2.5$ dust mass increase in going from MW diffuse to dense clouds. This would correspond to a factor up to $\simeq 7$ in terms of dust emissivity per H atom (Köhler et al., 2015).

4.2.1.2 Studies of the Magellanic Clouds

In nearby galaxies, studies of the local grain processing are difficult to conduct, as the emissivity variations are smoothed out by the mixing of dense and diffuse regions. Even when potential evolutionary trends are observed, their interpretation is often degenerate with other factors. The Magellanic clouds are the most obvious systems where this type of study can be attempted. The insights provided by depletion studies (cf. Sect. 2.2.3) show that there are clear variations of the fraction of heavy elements locked-up in dust, and these variations correlate with the density (Tchernyshyov et al., 2015; Jenkins & Wallerstein, 2017). Since the coagulation and the accretion of mantles lead to an increase of FIR emissivity (cf. Fig. 1.21; Köhler et al., 2015), we should expect emissivity variations in the Magellanic clouds. Indeed, Roman-Duval et al. (2017) studied the trends of gas surface density (derived from H I and CO) as a function of dust surface density (derived from the IR emission), in these galaxies. They found that the observed dustiness of the LMC increases smoothly by a factor of $\simeq 3$ from the diffuse to the dense regions. In the SMC, the same variation occurs, with a factor of $\simeq 7$. They argue that optically thick H I and CO-free H₂ gas (cf. Sect. 3.3.2.3) can not explain these trends, and that grain growth is thus the most likely explanation.

Spatially-resolved SED fitting of LMC-N$$44 and SMC-N$$66. We have conducted a similar study, focussing on two massive star-forming regions, rather than the whole galaxies ⁵: (i)  N$$44 in the LMC; and (ii) N$$66 in the SMC (Galliano, 2017). Our maps were 200 pc wide regions, with a spatial resolution of $\simeq 15$ pc. The MIR-to-submm data were coming from the SAGE/HERITAGE surveys (Spitzer and Herschel data; Meixner et al., 2006, 2013). We used the hierarchical Bayesian SED model, HerBIE, with the AC dust composition of Galliano et al. (2011, cf. Sect. 3.1.3.3). The goal was to perform a spatially-resolved modeling of the dust properties, in a region with a strong gradient of physical conditions, in order to probe dust processing, as a function of density, ISRF and metallicity. The wide range of physical conditions can be estimated by looking at the range of SEDs shown in Fig. 4.8. In both panels, the faintest pixels show a rather cold SED, peaking around $\lambda \simeq 100\mu m$, whereas the brightest pixels peak around $\lambda \simeq 60\mu m$, with a very broad FIR bump, indicating a wide range of ISRF intensities, typical of compact SF regions (cf. Sect. 3.1.2.2).

Derived dust-gas relations. We have compared the derived dust and total gas column densities. The latter was estimated from the H Iline and ${}^{12}$CO(J$=$1$\to$0)${}_{2.6\mathrm{mm}}$ measurements (Meixner et al., 2006; Gordon et al., 2011; Meixner et al., 2013). The results are displayed in Fig. 4.9. The orange line represents the Galactic dustiness (cf. Table 2.4) scaled by the metallicity, therefore representing the Galactic dust-to-metal mass ratio. This line corresponds to the values we would expect if the dust constitution was close to the diffuse ISM of the MW and was not evolving with density. The most diffuse pixels in both regions are consistent with this value. The hatched yellow area corresponds to a dustiness larger than the metallicity, that is requiring more heavy elements in dust than what is available in the ISM. Overall, the trends of Fig. 4.9 indicate a non-linear dust-to-gas relation, with a variation of the observed dustiness by a factor of $\simeq 3$, similar to the studies we have reviewed at the beginning of this section. The high density pixels lie in the forbidden zone. The possible causes are the following.

Grain growth:

a part of this trend is likely the result of the evolution of the true dustiness, due to mantle accretion in denser regions. The yellow hatched area can however be considered as a hard upper limit, as in practice, not all heavy elements are refractory: even in the densest molecular clouds, there are gas phase CO, HCN, etc. This sole factor is therefore probably not sufficient to explain the full extent of the observed dustiness variation.

Emissivity increase:

grain growth and coagulation are accompanied by an increase of FIR emissivity (cf. Fig. 1.21; Köhler et al., 2015). This effect is naturally expected and would amplify the increase of the observed dustiness with density, as the constant emissivity assumption of our SED model would lead us to overestimate the dust mass of dense regions.

Contribution of dark gas:

we have not accounted for CO-dark gas (cf. Sect. 3.3.2.3). This component can potentially bias the molecular gas mass estimate in translucent regions by up to a factor $\simeq 100$ (Madden et al., 2020). It could also explain a part of the trend, as it would result in an underestimate of the total gas mass. This effect should however be significative at intermediate column densities, and decrease toward the densest regions, where CO would dominate. It might thus not be the main cause of the non-linearity of our trends.

4.2.1.3 Quantifying Grain Growth

We now discuss the way grain growth can be approximately quantified. The following relations are rather uncertain, because of the lack of constraint on grain structure and composition. They however provide a framework to study grain growth efficiency.

Accretion timescale. Timescales for grains to accrete atoms are widely discussed in the literature (e.g. Dwek, 1998; Edmunds, 2001; Draine, 2009; Hirashita & Kuo, 2011; Zhukovska et al., 2016; Priestley et al., 2021). First, the collision rate of an atom E of mass $m_E$, with a grain of radius $a$ is:

In this equation, we have implicitly neglected Coulomb interaction (i.e. we have assumed that the grain and the atom are both neutral, which is a reasonable assumption in the CNM). Second, the growth rate of a grain of mass $m_d\left(a\right)$, due to accretion following these collisions, can be written:

where $0\le \mathcal{S}\lesssim 1$ is the sticking coefficient, that is the probability the atom will be bound with the grain after the collision. The factor $f_E$ is the mass fraction of element E within the grain. We choose E as a key element (Zhukovska et al., 2008), that is the element in the grain make-up that will have the longest collision time.

For silicates,

the key element is Si, with $f_{\text{Si}}\simeq 0.16$ for olivine and $f_{\text{Si}}\simeq 0.24$ for pyroxene (assuming Fe:Mg=1:1). In other words, for each collision with Si, there are more collisions with O, Fe and Mg. Therefore, the dust mass gained between two Si collisions is the mass of a full crystal unit (SiO${}_4$MgFe for olivine SiO${}_3$Mg${}_{0.5}$Fe${}_{0.5}$ for pyroxene; cf. Sect. 1.1.4.1).

For carbon grains,

the mass is essentially C, as H is negligible. We thus have $f_C\simeq 1$.

Finally, it is convenient to express this quantity as an accretion timescale, ${\tau }_{\mathrm{acc}}\left(a\right)$:

where we have simply developed $m_d\left(a\right)=4∕3\pi a^3\rho$ in the second equality, $\rho$ being the mass density of the grain. The density of the element E can be written as a function of the total H density, assuming its abundance scales with metallicity:

We therefore see that the grain growth timescale roughly obeys the following proportionality (assuming $\mathcal{S}=1$ and olivine composition of silicates):

As said above, these estimates are uncertain. We especially have no idea of the sticking probability, $\mathcal{S}$. Eq. ($4.19$) however provides a description of the sensitivity of grain growth to density, size and metallicity. It is also indicative of the lower limit of these timescales.

Grain growth in different ISM phases. Fig. 4.10 displays Eq. ($4.19$) for carbon and silicate grains in the most relevant ISM phases. Timescales longer than the typical destruction timescales by SN II blast waves are irrelevant. That is the reason why this range is hatched in yellow in Fig. 4.10.

In the WNM,

only the smallest grains ($a\lesssim 10$ nm) could grow. The WNM is thus not very suitable for grain growth.

In the CNM,

all grain sizes can grow in less than 300 Myr. The CNM is thus a phase where dust growth could happen.

In diffuse molecular clouds,

growth timescales are roughly similar to the CNM. The same conclusion therefore applies.

In dense molecular clouds,

all relevant interstellar grain sizes can grow in less than $\simeq 10$ Myr, which is also the typical lifetime of these clouds, in star-forming regions.

These timescales are consistent with the picture painted by the variation of elemental depletions across phases (cf. Fig. 2.17.a). To estimate a global growth timescale, let’s consider the radius corresponding to the average mass of the THEMIS model, in Table 2.3:

With these sizes, a typical accretion time in the CNM would be ${\tau }_{\mathrm{acc}}\simeq 58$ Myr for silicates, and ${\tau }_{\mathrm{acc}}\simeq 38$ Myr for large a-C(:H).

Relation to global parameters. As we will see in Sect. 4.3, it is convenient to relate the grain growth timescale to global galaxy parameters. Mattsson et al. (2012) proposed a relation based on the following assumptions.

1.

Most of grain growth happens in molecular clouds. The mass surface density of these molecular clouds, ${\Sigma }_{\text{mol}}$, is proportional to the SFR surface density, ${\Sigma }_{\mathrm{SFR}}$ (e.g. Kennicutt, 1998b). Grain growth rate is thus proportional to ${\Sigma }_{\mathrm{SFR}}$:

$$\frac{{\Sigma }_{\mathrm{gas}}}{{\tau }_{\mathrm{grow}}}\propto {\Sigma }_{\mathrm{SFR}}.$$

(4.21)

2.

The grain growth rate is also proportional to the fraction of available heavy elements in the gas. It implies that:

$$\frac{{\Sigma }_{\mathrm{gas}}}{{\tau }_{\mathrm{grow}}}\propto {\Sigma }_{\mathrm{SFR}}\times \left(1-\frac{Z_{\mathrm{dust}}}{Z}\right)Z,$$

(4.22)

where $Z_{\mathrm{dust}}$ is the dustiness, and $Z_{\mathrm{dust}}∕Z$, the dust-to-metal mass ratio (cf. Sect. 2.2.3.2). By subtracting $Z_{\mathrm{dust}}∕Z$, we account for the fact that the fraction of heavy elements already locked up in grains does not contribute to grain growth.

3.

The other parameters in Eq. ($4.19$) are assumed to not vary significantly. These parameters are the mean grain size, the mean gas velocity and mean density of molecular clouds.

The grain growth rate proposed by Mattsson et al. (2012) can thus be parametrized as a function of global galactic quantities and a phenomenological, dimensionless parameter, ${𝜖}_{\mathrm{grow}}$, containing all our uncertainties. The goal is to empirically infer ${𝜖}_{\mathrm{grow}}$, as we will see in Sect. 4.3. Eq. ($4.22$) thus becomes:

where we have replaced the ratio of surface densities by the ratio of the quantities, and have explicited the temporal dependencies. In the case of the MW ($\psi \simeq 1.3M_{\odot }$/yr; $M_{\mathrm{gas}}\simeq 7\times 10^9{M}_{\odot }$), a grain growth timescale of ${\tau }_{\mathrm{grow}}\simeq 60$ Myr (Eq. $4.19$) corresponds to ${𝜖}_{\mathrm{grow}}\simeq 10^4$.

4.2.2 Grain Destruction

We now discuss grain destruction, that is the return of heavy elements from the grains to the gas phase. Note that fragmentation and shattering by shock waves (at $v\lesssim 200$ km/s), that we have discussed in Sect. 4.2.2.3, simply rearrange the size distribution without destroying the dust. Shocks however have a pulverization effect, accompanying the other processes, that are difficult to differentiate from an observational point of view.

4.2.2.1 Photodestruction of Small Grains

Due to thermal spikes, small grains have a certain probability that one of their atom will be ejected. This is a runaway process leading to the complete sublimation of the dust grain.

Photodesorption and sublimation. Following the formalism of Guhathakurta & Draine (1989), we consider a cluster $X_N$ containing $N$ atoms of $X$ ($X$ can be C, Fe, Si, O, etc.). The ejection of an atom from the grain is balanced by the return of an atom from the gas phase. The rate of the reaction $X_N+X\leftrightarrows X_{N+1}$ is $R_N{A}_N$, where the total grain surface is $A_N=4{\sigma }_N\simeq 4\pi a^2$. Guhathakurta & Draine (1989) write the sublimation rate as:

and provide the following rates for graphite and silicate:

with the binding energy per atom:

The sticking coefficients, ${\alpha }_N$, is unknown and is arbitrarily chosen by the authors to be ${\alpha }_N\simeq 0.1$. Assuming the surface free energy is about $2\times 10^4$ K, the term $-20000N^{-1∕3}$ accounts for the surface tension, making it easier to release an atom when the grain is smaller. Finally, the suppression factor, $S_N\left(T\right)<1$, accounts for the suppression of the thermal fluctuations in a thermally isolated particle. This factor is:

where $f=3N-6$ is the number of vibrational degrees of freedom (cf. Sect. 1.2.3). In addition, the mean number of quanta per degree of freedom is $\gamma =H\left(T\right)∕\left(\hslash {\omega }_{0}f\right)$, and the number of quanta necessary to release a particle is $b=B∕\left(\hslash {\omega }_0\right)$. Guhathakurta & Draine (1989) take $\hslash {\omega }_0=0.75\Theta$, where $\Theta$ is the Debye temperature (taking ${\Theta }_{\mathrm{gra}}=420\text{K}$ and ${\Theta }_{\mathrm{sil}}=470\text{K}$; cf. Sect. 1.2.3.2). The mean lifetime is then integrated over the temperature distribution:

Guhathakurta & Draine (1989) assume that a grain does not survive if it has a lifetime $\lesssim 10^{13}\text{s}\simeq 0.3$ Myr. Fig. 4.11 displays these lifetimes for silicates and graphite bathed in the Mathis et al. (1983) ISRF. Although the exact numbers are to be taken with caution, we can conclude the following.

1.

In the diffuse ISM of the MW ($U=1$), silicates larger than $a\simeq 4.5$ Å and graphite $a\simeq 3.5$ Å can survive.

2.

When $a$ increases, the lifetimes become exponentially longer, meaning that for grains larger than $a\gtrsim 6$ Å, the survival of these grains will not be very sensitive to the assumptions in Eq. ($4.28$).

The hardness of the ISRF, that we have not represented here, will however increase the minimum size a grain needs to have in order to survive. The vicinity of OB associations will thus be environments where the smallest grains can be photodestroyed.

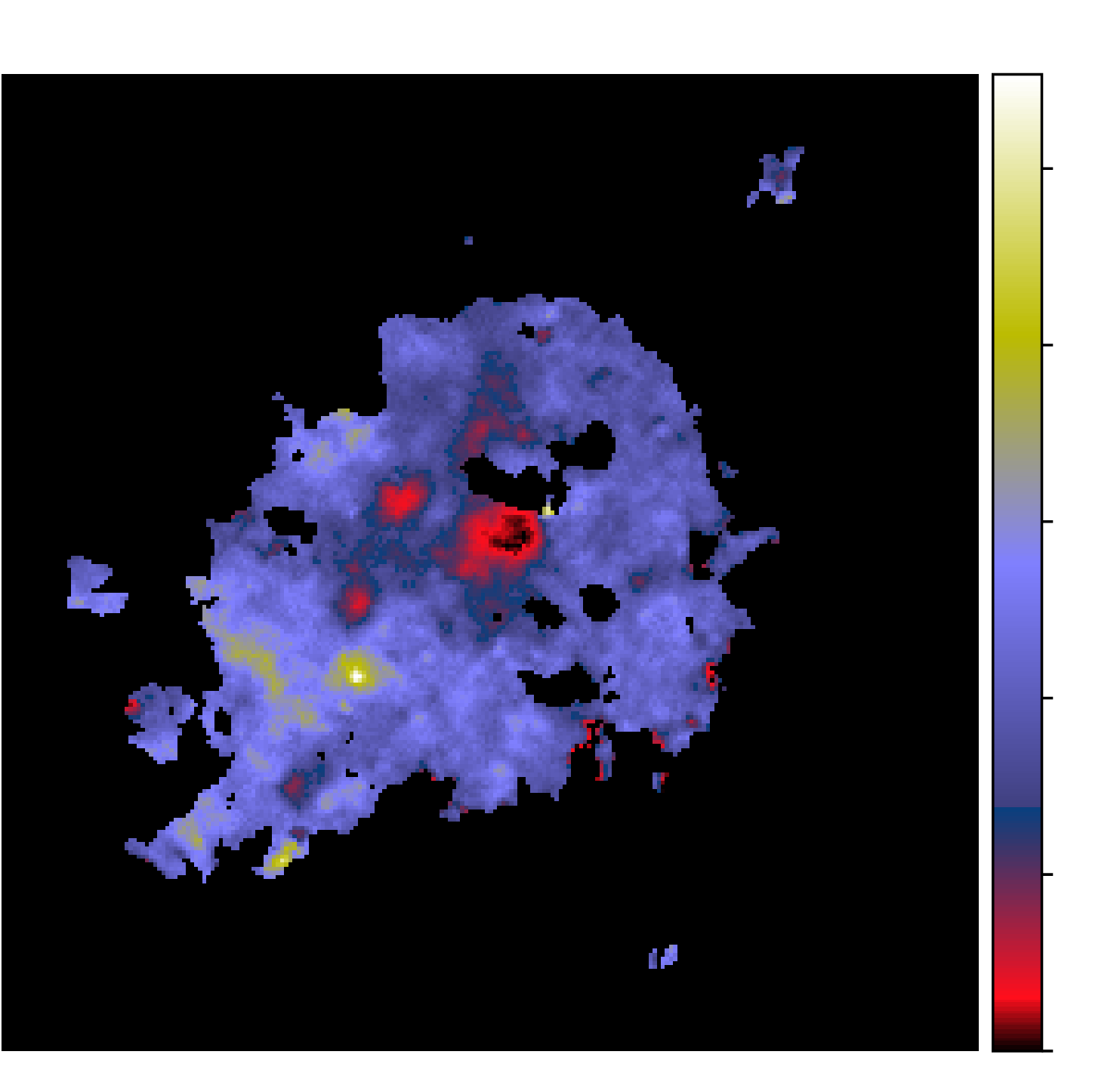
Evidence in resolved regions. This last point is observationally verified in countless regions. It can be conveniently witnessed, as the smallest grains are the carriers of the MIR continuum, which is well separated from the rest of the emission (cf. Fig. 2.27.b). In addition, small carbon grains carry the series of aromatic features (cf. Sect. 3.2.1.1). The disappearance of these features in regions of enhanced ISRF is very likely the sign of the destruction of these grains by hard UV photons. This is, for instance, evident in one of our studies of the massive star-forming region, N$$11, in the LMC (Galametz et al., 2016). This region contains several blobs, with embedded star clusters. The maps of the PAH mass fraction, $q_{\mathrm{PAH}}$ (cf. Sect. 3.1.2.2), is shown in Fig. 4.12.a. It has been derived by modeling the spatially-resolved SED of Spitzer and Herschel images. Comparing this image to the mean starlight intensity in Fig. 4.12.b, we see that PAHs are strongly depleted in the blobs where $⟨U⟩$ is enhanced. The photodestruction is evident. In the case of this massive region, a bright star cluster such as N$$11B can clear PAHs out over a region of typically $\simeq 50$ pc.

4.2.2.2 Thermal Sputtering

Another destruction mechanism is grain erosion and vaporization by collisions with energetic ions, either in coronal plasmas (thermal sputtering; $T\gtrsim 10^6$ K), or in shock waves (kinetic sputtering; $v\gtrsim 100$ km/s). There is an abundant literature on the subject (e.g. Draine & Salpeter, 1979; Dwek & Scalo, 1980; Tielens et al., 1994; Jones et al., 1996; Jones, 2004; Nozawa et al., 2006; Micelotta et al., 2010; Bocchio et al., 2012, 2014; Hu et al., 2019, see also the review by Dwek & Arendt, 1992). We start by discussing thermal sputtering in this section, and will review kinetic sputtering in Sect. 4.2.2.3.

Sputtering times. The evolution of a grain of radius, $a$, and mass, $m_d\left(a\right)=4∕3\pi a^3\rho$, subjected to sputtering in a gas of density $n_H$, can be expressed (e.g. Hu et al., 2019):

where we have hidden all the microphysics into the sputtering yield, $Y_{\mathrm{sput}}\left(T_{\mathrm{gas}},v_s\right)\equiv da∕dt∕n_H$. This quantity depends on: (i) the gas temperature, $T_{\mathrm{gas}}$, in case of thermal sputtering; or (ii) the shock velocity, $v_s$, in case of kinetic sputtering. A detailed derivation of $Y_{\mathrm{sput}}$ can be found in Nozawa et al. (2006, Sect. 5). For our simple discussion, we will adopt their yields, for silicate and carbon grains, fitted by Hu et al. (2019). In the thermal case, the sputtering rate can be expressed as:

Fig. 4.13.a shows the lifetimes of grains in a coronal plasma. With Eq. ($4.30$), in the HIM (cf. Table 3.6), typical grains (Eq. $4.20$) have lifetimes of: (i) ${\tau }_{\mathrm{sput}}^{\mathrm{th}}\simeq 11$ Myr, for silicates; and (ii) ${\tau }_{\mathrm{sput}}^{\mathrm{th}}\simeq 16$ Myr, for carbon grains. In the case of SN II blastwaves, grains stay in post-shock conditions for only $\simeq 10^4$ yr. Dust destruction by thermal sputtering is thus not the dominant process in the shocked ISM.

Early-type galaxies. We have seen in Sect. 3.1.3.1 that ETGs tend to be characterized by a diffuse X-ray emission, originating in a permeating coronal gas. This HIM is likely filling most of their ISM. This has consequences on the dust properties. This can be seen in Fig. 4.14.a, looking at a classic scaling relation between the dustiness and the specific gas mass ⁶, $s{M}_{\mathrm{gas}}\equiv M_{\mathrm{gas}}∕M_{\star }$. Most ETGs appear to be distributed on a vertical branch, below the main trend. They appear to be depleted in dust, at a given specific gas mass. Investigating the contribution of the X-ray emitting coronal gas, we have displayed the specific dust mass, as a function of the X-ray-luminosity-to-dust-mass ratio, $L_X∕M_{\mathrm{dust}}$, in Fig. 4.14.b (G21). The $L_X∕M_{\mathrm{dust}}$ ratio quantifies the X-ray photon rate per dust grain. We see that ellipticals occupy the lower right corner of this relation: they have a high photon rate per dust grain and a low specific dust mass. We have just shown that grains in a hot gas have a short lifetime (Eq. $4.30$). The correlation of Fig. 4.14.b is thus likely the result of enhanced thermal sputtering in ETGs.

4.2.2.3 Destruction by SN Blast Waves

We now focus on the effect of kinetic sputtering. This process leads to erosion and vaporization of grains in SN II blast waves. As we will see, this happens to be the major dust grain destruction mechanism. The kinetic sputtering rate is similar to the thermal case (Eq. $4.30$), except that the sputtering yield now depends on the shock velocity, $v_s$ (cf. Fig. 4.13.b):

In addition, grain shattering in grain-grain collisions is an important dust destruction mechanism in SN II blast waves (e.g. Kirchschlager et al., 2021).

Evidence in resolved regions. Although the efficiency of the process is debated, the reality of dust destruction by SN II shock waves is rather consensual. This process can even be observed in spatially-resolved SNRs. In particular, ISO and Spitzer MIR spectra of pre-shock and post-shock matter show systematic differences in, for instance: (i)  3C$$391 (Reach et al., 2002); (ii)  SN$$1987A (Dwek et al., 2008; Arendt et al., 2016); and (iii) Puppis$$A (Arendt et al., 2010). The post-shock ISM exhibits:

• the disappearance of the aromatic features;
• the disappearance of the dust continuum.

Global model prescription. Similarly to what we did for grain growth (Eq. $4.23$), it is convenient to express the dust destruction rate as a function of global galactic quantities. Such a formula was proposed by Dwek & Scalo (1980):

where $R_{\mathrm{SN}}\left(t\right)$ is the SN II rate (Eq. $4.11$), and $m_{\mathrm{gas}}^{\mathrm{dest}}$ is an empirical parameter quantifying the destruction efficiency. The latter represents the gas mass swept by a single SN II blast wave, within which all grains are destroyed. Eq. ($4.32$) can be understood the following way.

1.

A single SN II destroys a mass $Z_{\mathrm{dust}}\times m_{\mathrm{gas}}^{\mathrm{dest}}$ of dust.

2.

Knowing the SN II rate, the dust mass destroyed per unit time is therefore:

$${\left(\frac{d{M}_{\mathrm{dust}}}{dt}\right)}_{\mathrm{dest}}=Z_{\mathrm{dust}}{m}_{\mathrm{gas}}^{\mathrm{dest}}\times R_{\mathrm{SN}}\left(t\right).$$

(4.33)

3.

The dust destruction rate is then simply $1∕{\tau }_{\mathrm{SN}-\mathrm{dest}}={\left(d{M}_{\mathrm{dust}}∕dt\right)}_{\mathrm{dest}}∕M_{\mathrm{dust}}$, which gives Eq. ($4.32$).

The destruction efficiency. The dust destruction efficiency, quantified by the parameter $m_{\mathrm{gas}}^{\mathrm{dest}}$ in Eq. ($4.32$), ranges in the literature between $\simeq 100M_{\odot }$ and $\simeq 1000M_{\odot }$. It can be roughly estimated with the following arguments (Draine, 2009, slightly adapting his numbers):

1.

In a ISM of density $n_H\simeq 1$ cm${}^{-3}$, a SN II, with energy $E_0\simeq 10^{43}$ J ($10^{51}$ erg), produces a blast wave that stays in the Sedov-Taylor phase (adiabatic expansion), until reaching a velocity $v_s\simeq 200$ km/s (Eq. 39.22 of  Draine, 2011).

2.

Grains are primarily destroyed in the Sedov-Taylor phase. At the end of this phase, the radius of our blast wave is $R_{\mathrm{Sedov}}\simeq 24$ pc (Eq. 39.21 of  Draine, 2011), corresponding to a total gas mass of $M_{\mathrm{Sedov}}\simeq 1900M_{\odot }$, which is a rough estimate of our efficiency parameter, $m_{\mathrm{gas}}^{\mathrm{dest}}$.

3.

At solar metallicity (using the dustiness of Table 2.4), this corresponds to $M_{\mathrm{dust}}^{\mathrm{dest}}\simeq 10M_{\odot }$.