Prediction of nuclear properties based on a realistic description of the strong interaction is at the heart of the ab initio endeavor in low-energy nuclear theory. Ab initio calculations have long been limited to light nuclei or to nuclei with specific proton and neutron numbers. Theoreticians from Irfu/DPhN have developed a new ab initio method from which properties of many more nuclei than before can be predicted while drastically decreasing the computational cost. This has been made possible by allowing symmetries of the nuclear Hamiltonian to spontaneously break in the calculation. This exciting new development, paving the way for precise computations of heavier nuclei within a reasonable time-frame, has recently been published in Physics Letter B [1].
Atomic nuclei are systems composed of nucleons, the protons and neutrons, interacting via inter-nucleon forces. The interactions between nucleons result from strong interactions between quarks and gluons that constitute them, the framework of which is provided by the quantum field theory of Quantum Chromo Dynamics (QCD). Unfortunately, QCD cannot be applied at the energy characterizing phenomena displayed by the atomic nucleus. In this context, how can one describe atomic nuclei from first principles in a systematic and controllable way? This longstanding (and still unanswered) question is at the heart of the so-called ab initio approach to the nuclear quantum many-body problem. It requires i) to model the inter-nucleon interactions entering the A-body Schrödinger equation (A is the number of nucleons composing the nucleus) with a sound connection to QCD and ii) to develop mathematical methods allowing for accurate and controlled approximations of the exact solutions of the A-body Schrödinger equation.
Three decades ago, the seminal work of S. Weinberg paved the way for a systematic theory of inter-nucleon interactions anchored into QCD. Nuclear Hamiltonians1 designed within this framework have nowadays become the standard input to the A-body Schrödinger equation. Having nuclear interactions with predictive power available led — over the years — to the subsequent replacement of former phenomenological interactions like the so-called Argonne or Nijmegen ones. However, even with state-of-the-art nuclear Hamiltonians at hand, finding the solution of the Schrödinger equation for a large range of nuclei constitutes a highly non-trivial problem, both from a formal and a computational perspective, such that calculations have long been limited to light systems with mass number A ≤ 12. Over the past 15 years, mathematical methods expanding the exact solution with respect to a simple reference state have been designed and have made the description of heavier nuclei up to tin isotopes possible. However, these methods have remained limited until recently to nuclei with specific numbers of protons and neutrons: the so-called doubly closed-shell nuclei. Indeed, to first approximation, a nucleus can be described by a state obtained by filling up protons and neutrons on two sets of shells that can only accept a specific number of them each. When the proton and neutron numbers are such that the upper neutron and proton shells are entirely filled, the corresponding nucleus is coined as “doubly closed-shell”. These nuclei are relatively more stable and simpler to describe than their neighbors as they authorize the use of standard Slater determinants as reference states. In the past years, theoreticians from Irfu/DPhN have developed three different expansion methods allowing one to perform ab initio calculations of singly open-shell nuclei; i.e. nuclei whose upper proton or neutron shell is not fully occupied and that are relatively more challenging to solve for. This has extended the reach of ab initio calculations from a few tens to several hundreds of nuclei.
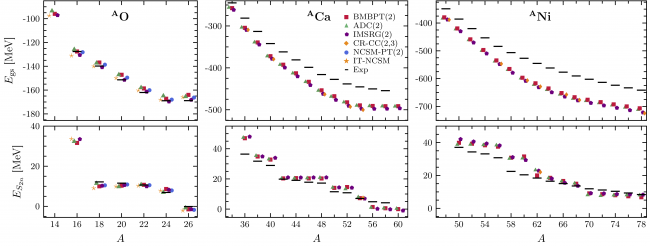
Figure 1: Ground-state binding energies (top) and two-neutron separation energies (bottom) along O, Ca and Ni isotopic chains. Results using other many-body methods are shown for comparison. Experimental values are shown as black bars.
The key idea behind these approaches is to allow the reference state to break a symmetry of the underlying Hamiltonian. For semi-magic nuclei, the relevant symmetry to be broken is the so-called U(1) global gauge symmetry, an abstract symmetry associated with the simple fact that nuclei are made of specific numbers of protons and neutrons. In these approaches, the system is first allowed to not have exactly Z protons or N neutrons in order to handle the complexity associated with the partially filled character of the upper shell. This idea leads to employing a so-called Bogoliubov reference state (solving the Hartree-Fock-Bogoliubov mean-field equations) that generalizes the use of a simpler Slater determinant (solution of the well-celebrated Hartree-Fock mean-field theory), which allows to capture from the outset the superfluid character of singly open-shell nuclei. While the breaking of U(1) symmetry is a standard tool in simple mean-field descriptions, it has never been applied in beyond mean-field methods aiming at an accurate solution of the A-body Schrödinger equation and thus allowing for ab initio calculations. The latest of the three formalisms developed by theoreticians from Irfu/DPhN consists of a perturbative expansion around the particle-number-breaking Bogoliubov reference state and is thus coined as Bogoliubov many-body perturbation theory (BMBPT). In Fig. 1, a systematic comparison of BMBPT results with other state-of-the-art methods, among which one has also been developed by the same group (ADC(2)), is shown for three different isotopic chains. While it is obvious that BMBPT performs extremely well against existing methods for both binding energies and two-neutron separation energies, it does so for a computational price that is two orders of magnitude lower. This makes BMBPT an extremely useful candidate for performing large survey calculations across the nuclear chart and makes the future extension to the even more challenging doubly open-shell nuclei simpler than in other frameworks.
In summary, the theoreticians from Irfu/DPhN have added a new ab initio quantum many-body method dedicated to the ab initio description of mid-mass open shell nuclei that can compete with all previously available method at a much lower computational cost. Being almost entirely developed at Irfu/DPhN, this newly-designed method marks the increasing significance of the CEA theory group in the sector of ab initio nuclear structure theory.
1. Nuclear Hamiltonian: mathematical operator describing the dynamics of A interacting nucleons. In the case of the nuclear Hamiltonian, it is written as the sum of the kinetic energies of the A nucleons and the sum of the 2-body, 3-body… interactions between the nucleons.
[1] A. Tichai, P. Arthuis, T. Duguet, H. Hergert, V. Somà, R. Roth, Phys. Lett. B786 (2018) 195.
